Developing Triangle and Quadrilateral Area

I have seen and thought a lot recently about the development for approaches to calculating area of certain polygons, particularly triangles and quadrilaterals. I haven't had much time for blogging as exams and end of year routine have dominated my waking hours however now I have some space I thought I would take the time to summarise what I have seen/thought about/heard.

Early development

I think it is fairly standard practice when introducing area calculations to start with the square and rectangle. The use of shapes on a square grid (like the examples below) to motivate the calculation of area as the square of length, or the product of length and width:

Here is where I see typically see the first deviations in teaching approaches. Some people will move from the rectangle to the triangle, using rectangles to justify why the triangle area is halved - images like the ones below are typical (in fact, taken from my own lessons, but I will be revising my approach when I teach to Year 7 next year).

Parallelograms would then follow, or be taught alongside; either as a tilted rectangle, or as a rectangle with a triangle removed and replaced as per the image below:

I was also shown this lovely image of a pile of books demonstrating the idea of rectangle and parallelogram areas being equal at the most recent Complete Mathematics Conference.

One approach I saw recently, which I believe has real merit, is to secure understanding of parallelogram area before moving onto triangle area. This is the approach I will be using from next year for one big reason - the obtuse-angled triangle. Whilst the rectangle can demonstrate right and acute-angled triangles, it is impossible to demonstrate that the area of the obtuse-angled triangle is

½ x base x height, or even adequately show what the base and height of an obtuse-angled triangle are. 

However if one is secure in parallelogram area calculation, and secure in the idea that a rectangle is a parallelogram with extra-properties, then using parallelograms to demonstrate triangle areas deals nicely with obtuse, acute and right-angled triangles. The images below make this clear:

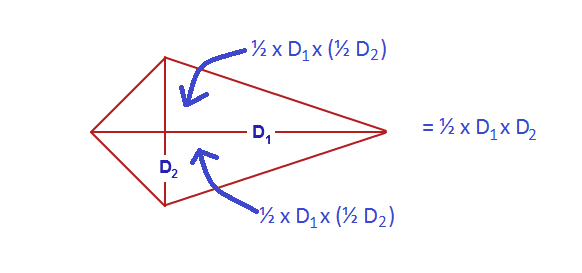
It has always struck me as somewhat odd here that the only other shape for which knowledge of the area formula is required is the trapezium. It would seem perfectly logical to me to move on from the parallelogram to teach the area of the kite - particularly as this can also be seen as two triangles (although this is also perhaps why it isn't taught, as it can be broken nicely into two triangles)...

I would advocate strongly for this to be included when developing the idea of area as the kite, together with the rectangle is a lovely way to then discuss the duality of approach that is available when calculating the area of a rhombus.

Most pupils will have little difficulty in seeing the relationship between a parallelogram and a rhombus. Slightly less intuitive is the relationship between a rhombus and a kite. Orientation can help; having a rhombus standing on a vertex rather than an edge makes the link more visible. It is a lovely meeting point for areas covered so far, as well as enriching pupils' understanding of rhombi...

Of course then from the area of a rhombus and kite and come the area of an arrowhead...

For me, this is then the point to move onto the trapezium. There are a huge number of ways of deriving the calculation for the area of a trapezium, and although the major ones only require knowledge of rectangles, parallelograms and triangles, the manipulation of areas used in deriving the calculation for a kite, rhombus and arrowhead should be useful preparation work. We spent some time at a recent meeting looking at lots of different ways to calculate the area of a trapezium. Here are some of my favourites:

Trapezium as the sum of two triangles

Trapezium as half a parallelogram

Trapezium turned into a parallelogram

Trapezium turned into a rectangle

Trapezium turned into a triangle

Trapezium as a rectangle and a triangle

Trapezium as a parallelogram and a triangle

The benefit of exploring these different approaches is in the richness of understanding of both area calculations and algebraic manipulation that can be developed with a carefully structured teaching approach. This is definitely what I will be aiming to do with my teaching of area in the coming academic year.